Complex Patterns in Oscillatory Systems
 |
 |
 |
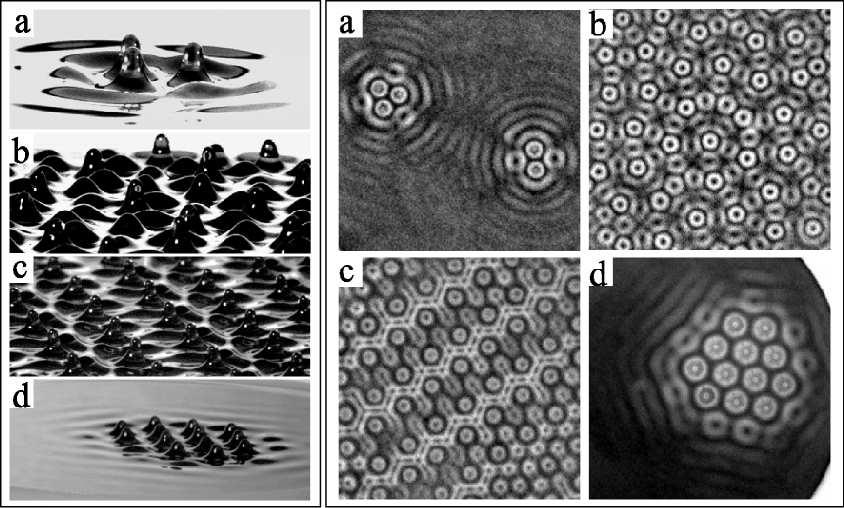
I am interested in understanding the formation of complex spatial patterns in oscillatory media with resonant forcing. Such periodic or quasi-periodic patterns have been studied and observed experimentally in Faraday systems where a thin liquid layer is vibrated vertically to form surface wave patterns like the superlattice patterns below.
 |
 |
An example of an oscillatory system is the Belousov-Zhabotinsky (B-Z) reaction, where oscillations are in chemical concentration. Experimental observations of the reaction in a thin layer have shown that the oscillations form travelling waves which can organize themselves into spiral waves. With the application of resonant forcing, the oscillations instead form standing waves which organize themselves in labyrinthine patterns. On the right is a snapshot from an experiment showing both regimes.
The universal description of small amplitude oscillations is given by the complex Ginzburg-Landau equation (CGLE) for the amplitude of oscillations. I extend this equation to include terms that describe external forcing near integer multiples of the natural or resonant frequency of oscillation of the system, where A represents the complex amplitude of oscillations:
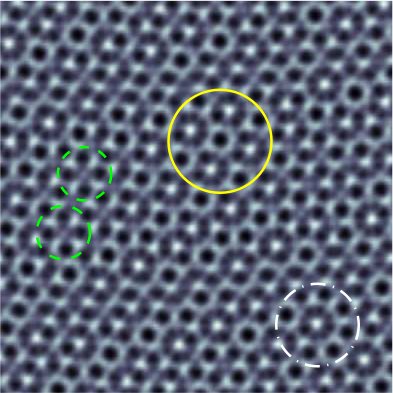
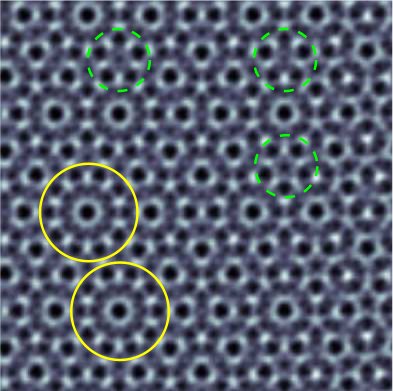
Such forcing induces the formation of spatial patterns in the system through the combination of standing waves of different orientations. I am interested in complex periodic or quasi-periodic patterns, which are formed by the combination of more than three waves. I have shown that in oscillatory systems patterns with up to 4-fold and 5-fold rotational symmetries can be made stable through suitable choice of the forcing function. In regimes where there is competition between stable patterns, I have used energy arguments to predict the pattern that will prevail. I then confirmed my predictions through numerical simulations in large systems. Below are snapshots from numerical simulations of the CGLE movies showing some of the different patterns; click on the images to see a movie of their temporal evolution from a noisy initial condition [6].
 |
 |
 |
 |
References:
[1] http://www.geniuswebs.co.uk/sublyme/clubweb/receipes/mackerell/Mackerel.jpg
[2] http://www.flatrock.org.nz/topics/environment/assets/altocumulus.jpg
[3] http://wallpaper.travelblog.org/Wallpaper/pix/tb\_mui\_ne\_sand\_dunes.jpg
[4] H. Arbell and J. Fineberg. Pattern formation in two-frequency forced parametric waves.
[5] V. Petrov, Q. Ouyang, and H. L. Swinney. Resonant pattern formation in a chemical system.
[6] J.M. Conway and H. Riecke. Quasi-patterns in a model of chemical oscillations with multiresonant forcing.